In the Figure Below, Triangle Abc Is Similar to Triangle Pqr: What Is the Length of Side Pq?
Congruence of triangles: Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal in measure out. These triangles can be slides, rotated, flipped and turned to be looked identical. If repositioned, they coincide with each other. The symbol of congruence is' ≅'.
The respective sides and angles of congruent triangles are equal. There are basically iv congruency rules that proves if two triangles are congruent. Merely information technology is necessary to find all vi dimensions. Hence, the congruence of triangles can be evaluated past knowing merely 3 values out of vi. The meaning of congruence in Maths is when two figures are similar to each other based on their shape and size. Also, acquire about Congruent Figures hither.
Congruence is the term used to define an object and its mirror image. Two objects or shapes are said to be coinciding if they superimpose on each other. Their shape and dimensions are the same. In the case of geometric figures, line segments with the same length are congruent and angles with the aforementioned measure are congruent.
CPCT is the term, we come across when we learn well-nigh the congruent triangle. Let's come across the condition for triangles to exist congruent with proof.
Coinciding significant in Maths
The pregnant of congruent in Maths is addressed to those figures and shapes that tin be repositioned or flipped to coincide with the other shapes. These shapes can be reflected to coincide with similar shapes.
2 shapes are congruent if they take the same shape and size. Nosotros can also say if two shapes are congruent, and so the mirror image of one shape is same as the other.
Congruent Triangles
A polygon made of three line segments forming iii angles is known as a Triangle.
2 triangles are said to be congruent if their sides have the aforementioned length and angles accept same measure. Thus, two triangles can be superimposed side to side and angle to angle.
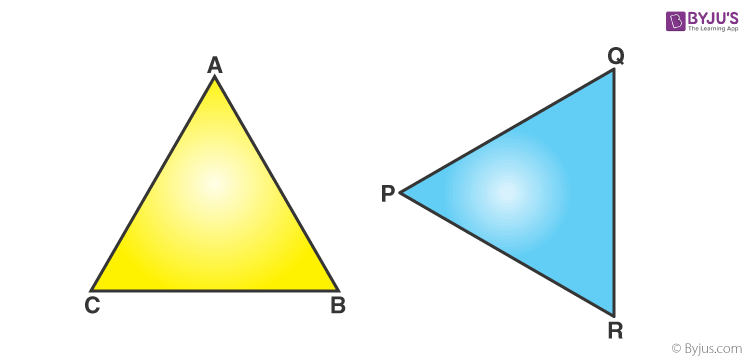
In the above figure, Δ ABC and Δ PQR are congruent triangles. This means,
Vertices: A and P, B and Q, and C and R are the aforementioned.
Sides: AB=PQ, QR= BC and Air-conditioning=PR;
Angles: ∠A = ∠P, ∠B = ∠Q, and ∠C = ∠R.
Coinciding triangles are triangles having corresponding sides and angles to be equal. Congruence is denoted by the symbol "≅". They have the same area and the same perimeter.
For More than Information On Introduction To Congruent Triangles, Watch The Beneath Video:
CPCT Total Form
CPCT is the term we come up across when nosotros larn about the congruent triangle. CPCT means "Corresponding Parts of Congruent Triangles". As nosotros know that the corresponding parts of coinciding triangles are equal. While dealing with the concepts related to triangles and solving questions, we ofttimes make utilise of the abridgement cpct in short words instead of full form.
CPCT Rules in Maths
The full form of CPCT is Corresponding parts of Congruent triangles. Congruence can be predicted without really measuring the sides and angles of a triangle. Different rules of congruency are as follows.
- SSS (Side-Side-Side)
- SAS (Side-Angle-Side)
- ASA (Angle-Side-Angle)
- AAS (Angle-Angle-Side)
- RHS (Right bending-Hypotenuse-Side)
Let u.s.a. learn them all in detail.
SSS (Side-Side-Side)
If all the three sides of one triangle are equivalent to the corresponding three sides of the second triangle, then the ii triangles are said to be congruent by SSS rule.
In the above-given effigy, AB= PQ, QR= BC and AC=PR, hence Δ ABC ≅ Δ PQR.
SAS (Side-Bending-Side)
If any two sides and the angle included betwixt the sides of one triangle are equivalent to the corresponding 2 sides and the bending between the sides of the 2d triangle, so the two triangles are said to be congruent by SAS rule.
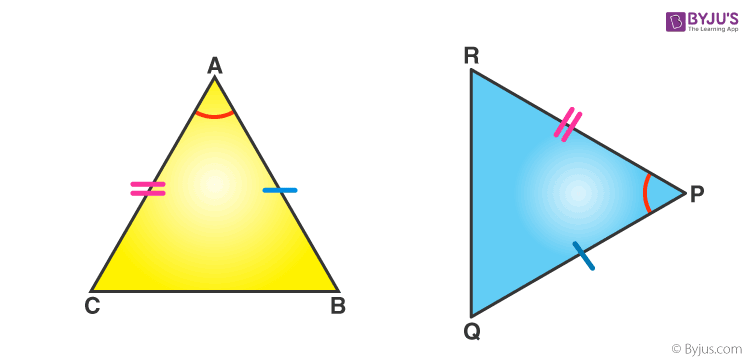
In above given figure, sides AB= PQ, AC=PR and angle between Ac and AB equal to angle between PR and PQ i.e. ∠A = ∠P. Hence, Δ ABC ≅ Δ PQR.
ASA (Angle-Side- Angle)
If any two angles and the side included between the angles of one triangle are equivalent to the respective ii angles and side included between the angles of the second triangle, then the two triangles are said to exist congruent by ASA rule.
In above given figure, ∠ B = ∠ Q, ∠ C = ∠ R and sides between ∠B and ∠C , ∠Q and ∠ R are equal to each other i.e. BC= QR. Hence, Δ ABC ≅ Δ PQR.
For More Information On SAS And ASA Congruency Rules, Spotter The Below Video:
AAS (Angle-Angle-Side) [Awarding of ASA]
AAS stands for Angle-angle-side. When two angles and a not-included side of a triangle are equal to the corresponding angles and sides of another triangle, and then the triangles are said to exist coinciding.
AAS congruency tin can be proved in easy steps. Suppose we have two triangles ABC and DEF, where,
∠B = ∠E [Corresponding sides] ∠C = ∠F [Corresponding sides] And
Air-conditioning = DF [Adjacent sides]
By bending sum belongings of triangle, we know that;
∠A + ∠B + ∠C = 180 ………(ane)
∠D + ∠E + ∠F = 180 ……….(2)
From equation 1 and two nosotros can say;
∠A + ∠B + ∠C = ∠D + ∠E + ∠F
∠A + ∠E + ∠F = ∠D + ∠E + ∠F [Since, ∠B = ∠E and ∠C = ∠F] ∠A = ∠D
Hence, in triangle ABC and DEF,
∠A = ∠D
AC = DF
∠C = ∠F
Hence, by ASA congruency,
Δ ABC ≅ Δ DEF
RHS (Right angle- Hypotenuse-Side)
If the hypotenuse and a side of a correct- angled triangle is equivalent to the hypotenuse and a side of the second right- angled triangle, so the two right triangles are said to be congruent by RHS dominion.
In in a higher place figure, hypotenuse XZ = RT and side YZ=ST, hence triangle XYZ ≅ triangle RST.
Solved Example
Example 1: In the following figure, AB = BC and Advertizing = CD. Show that BD bisects Air-conditioning at right angles.
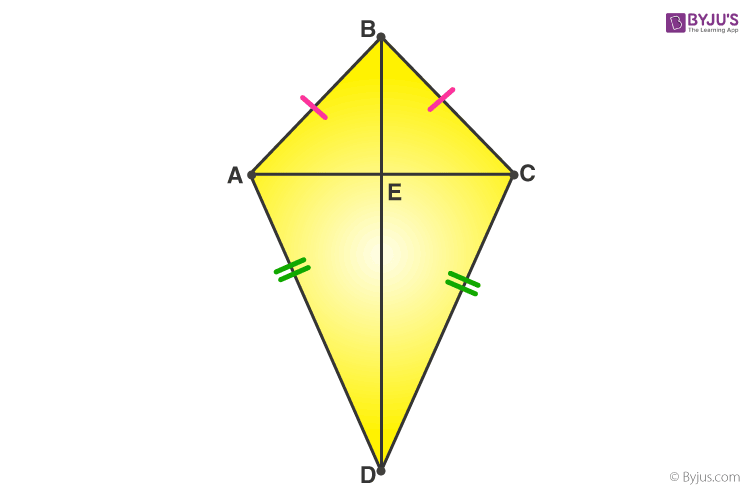Solution: We are required to testify ∠BEA = ∠BEC = xc° and AE = EC.Consider ∆ABD and ∆CBD,AB = BC (Given)Advertising = CD (Given) BD = BD (Common)
Therefore, ∆ABD ≅ ∆CBD (Past SSS congruency)
∠ABD = ∠CBD (CPCTC)
Now, consider ∆ABE and ∆CBE,
AB = BC (Given)
∠ABD = ∠CBD (Proved in a higher place)
Exist = Be (Common)
Therefore, ∆ABE≅ ∆CBE (By SAS congruency)
∠BEA = ∠BEC (CPCTC)
And ∠BEA +∠BEC = 180° (Linear pair)
2∠BEA = 180° (∠BEA = ∠BEC)
∠BEA = 180°/ii = 90° = ∠BEC
AE = EC (CPCTC)
Hence, BD is a perpendicular bisector of Air-conditioning.
Example ii: In a Δ ABC, if AB = AC and ∠ B = 70°, notice ∠ A.
Solution: Given: In a Δ ABC, AB = AC and ∠B = seventy°
∠ B = ∠ C [Angles opposite to equal sides are equal]
Therefore, ∠ B = ∠ C = 70°
Sum of angles in a triangle = 180°
∠ A + ∠ B + ∠ C = 180°
∠ A + 70° + 70° = 180°
∠ A = 180° – 140°
∠ A = forty°
Practice Problems
Q.1: PQR is a triangle in which PQ = PR and is any indicate on the side PQ. Through S, a line is drawn parallel to QR and intersecting PR at T. Prove that PS = PT.
Q.ii: If perpendiculars from whatever point within an angle on its arms are coinciding. Evidence that it lies on the bisector of that bending.
Video Lesson
For more details visit and register with us at BYJU'Due south. Also, download BYJU'S-The Learning App to relish personalised learning.
Often Asked Questions
What are Congruent Triangles?
Two triangles are said to be coinciding if the iii sides and the three angles of both the angles are equal in any orientation.
What is the Full Form of CPCT?
CPCT stands for Corresponding parts of Coinciding triangles. CPCT theorem states that if 2 or more than triangles which are congruent to each other are taken so the corresponding angles and the sides of the triangles are also coinciding to each other.
What are the Rules of Congruency?
At that place are 5 main rules of congruency for triangles:
- SSS Criterion: Side-Side-Side
- SAS Criterion: Side-Angle-Side
- ASA Criterion: Angle-Side- Bending
- AAS Benchmark: Angle-Angle-Side
- RHS Criterion: Correct angle- Hypotenuse-Side
What is SSS congruency of triangle?
If all the three sides of one triangle are equivalent to the corresponding three sides of the second triangle, so the ii triangles are said to be coinciding past SSS rule.
What is SAS congruence of triangles?
If any two sides and bending included betwixt the sides of one triangle are equivalent to the corresponding ii sides and the angle betwixt the sides of the second triangle, so the two triangles are said to be congruent by SAS dominion.
What is ASA congruency of triangles?
If whatever two angles and side included between the angles of one triangle are equivalent to the corresponding ii angles and side included betwixt the angles of the second triangle, then the ii triangles are said to be coinciding by ASA rule.
What is AAS congruency?
When ii angles and a not-included side of whatsoever two triangles are equal and so they are said to be congruent.
What is RHS congruency?
If the hypotenuse and a side of a right- angled triangle is equivalent to the hypotenuse and a side of the 2nd right- angled triangle, then the two correct triangles are said to exist congruent past RHS rule.
Source: https://byjus.com/maths/congruence-of-triangles/
0 Response to "In the Figure Below, Triangle Abc Is Similar to Triangle Pqr: What Is the Length of Side Pq?"
Post a Comment